The TED-Ed Lesson “The case of the missing fractals” isn’t just an introduction to the intricate and beautiful world of fractal geometry; it’s also a fully-realized film noir short complete with plot, drama and a smattering of ridiculous puns. This cinematic creation is the product of a TED-Ed dream team, with writing by veteran TED-Ed Educators George Zaidan and Alex Rosenthal (watch their lesson “Exploring other dimensions“) and animation by our own TED-Ed Animation Producer Jeremiah Dickey (watch his lesson, “A brief history of religion in art“). We met up with this trio to talk about the process of approaching an educational lesson with a narrative eye.
Why did you want to cover fractals? How did you come up with the idea for this lesson?
Alex Rosenthal: I started working on this when the previous lesson that George and I worked on together, which was about Flatland, premiered. A lot of our thinking was informed by what we felt worked with that one, because it also used really bizarre, mind-blowing geometry. We talked about a number of different ideas, and we picked fractals because it was another topic where we find a bizarre revelation about how the world works by diving into geometry. Plus, there is the opportunity to create really beautiful visuals for fractals. They really shine when you look at them, so they were a natural hook for the visual medium.
How did you decide you wanted to make this into a narrative piece, as opposed to a more straightforward lesson?
AR: I go in looking for the narrative. My background is in theater and in film, and I’m always looking for those ways to enter in that way, especially when it comes to math and science. Not just reporting the facts, but telling a story. I think a good story—and good characters—can help get you in and give you an empathetic, intuitive sense of the subject more than if it was just pure information.

For this lesson, educators George and Alex dreamed up the idea of a “fractal femme fatale.” Here, she appears in the lesson.
Why did you choose film noir for the genre?
George Zaidan: Well, Alex did write a non-noir version. He wrote a straight expository version of the fractals script, and then later he sent me this email saying, “I was in the shower, and I had an idea for how we might do this narratively.” Then he wrote the noir version of it, and it was just so fun. There were so many Easter eggs for the math and the noir genre that we both just were like, “Wow. This is great. This is really working.”
AR: Noir has a really beautiful sense of using language. The noir narrative style is very reliant on puns, and there are a lot of double and triple meanings. That appealed to me—it seemed a nice parallel to the structure of fractals. You know—how the deeper you go into them, the more you find the same thing, or you find layers upon layers that are almost tied to the same thing. The density of the language and the density of the geometry felt connected in a way that seemed to really work.
Jeremiah, you handpicked this lesson to work on. What made you want to animate it?
Jeremiah Dickey: The lesson was just written so well that the first time I read it, the story played out in my head almost exactly how it turned out on the screen. All except the fractals—those I had to research to actually wrap my head around. But I really loved the script that George and Alex wrote, and just wanted to see it done right. Also, cinephile that I am, I have a love for film noir that goes all the way back to film school, but I’ve never had a real opportunity to work in the genre. So, I jumped at the chance.
Alex and George, what did you think of the animation?
AR: Part of what I loved—something Jeremiah really brought to it—is that there’s something fundamentally otherworldly about fractals and yet very much of this world. The history of fractals is that they were actually a way of understanding nature better. You find fractals all over nature because nature works in the same way fractals do, in that they adhere to a set of rules that tend to iterate all the way down, repeatedly, until you get these beautiful, massive, infinite symmetries that you see everywhere from ferns to rivers to trees. The style that Jeremiah brought to it has a very fine, nuanced sense that captures the tension between Platonic geometry and fractal geometry—the geometry of order and the geometry of chaos. I think that really comes out in the mood and the environment that Jeremiah created.

In the lesson, a private eye must solve three riddles—each with a fractal for a solution.
It sounds like you all knew quite a bit about noir before diving into it. Did you have to do any additional research when you decided this was going to be the direction?
AR: I jumped into some noir dictionaries. I think getting the use of metaphor right is one of the main tells of noir, and so I read a lot of examples of that. George, did you watch The Maltese Falcon while you—
GZ: I absolutely watched The Maltese Falcon. I watched that, and—I haven’t told anyone this yet—but I said, “Well, look, I really want to do the voice because this is really different and new.” I was going to do the voice for Manny, and then either Alex or Jeremiah was going to do the voice for Sierpinski. But in the end, for travel reasons, I contributed both voices. Before we had decided, I took an acting class with my buddy, who told me, “All right, here’s the movies you need to watch. Here’s a radio story you need to listen to.” And we did an hour with Alex’s script, figuring out how to make it really sound like a noir detective.
I had no idea that you read both of the roles. So, great work! Jeremiah, what was your research process?
JD: My process usually involves soaking up as much information and visual references as I can about the subject matter without thinking too much about it, and then just seeing what comes out. True film noir usually has an element of weirdness that sets it apart from the more typical crime drama of the era, and the roots of that really come from German expressionism, brought over to Hollywood by German filmmakers fleeing Europe in the years leading up to WWII. So with this in mind, I spent time looking at German artists like Otto Dix, Käthe Kollwitz, George Grosz. Also American comic book artists of the era who did pulp/crime comics—like Mort Meskin and Alex Toth. I also watched a lot of films, like two or three a night, including many shot by a cinematographer named John Alton. There are also some homages to other films in there. One not so subtle one is Alfred Hitchcock’s Vertigo, which isn’t strictly a noir film, but it’s one of my favorites.
I also read up on Benoît B. Mandlebrot, the late revolutionary mathematician who coined the term fractal. The main character of the lesson is named for him. There is a great documentary on Mandlebrot and his work called The Colors of Infinity, which you can find on YouTube. I also got my hands on a copy of Mandlebrot’s book The Fractal Geometry of Nature. I wish I could say I read it, but I didn’t. I did study the pictures intensely, though, and used quite a few of them at the end of the lesson.
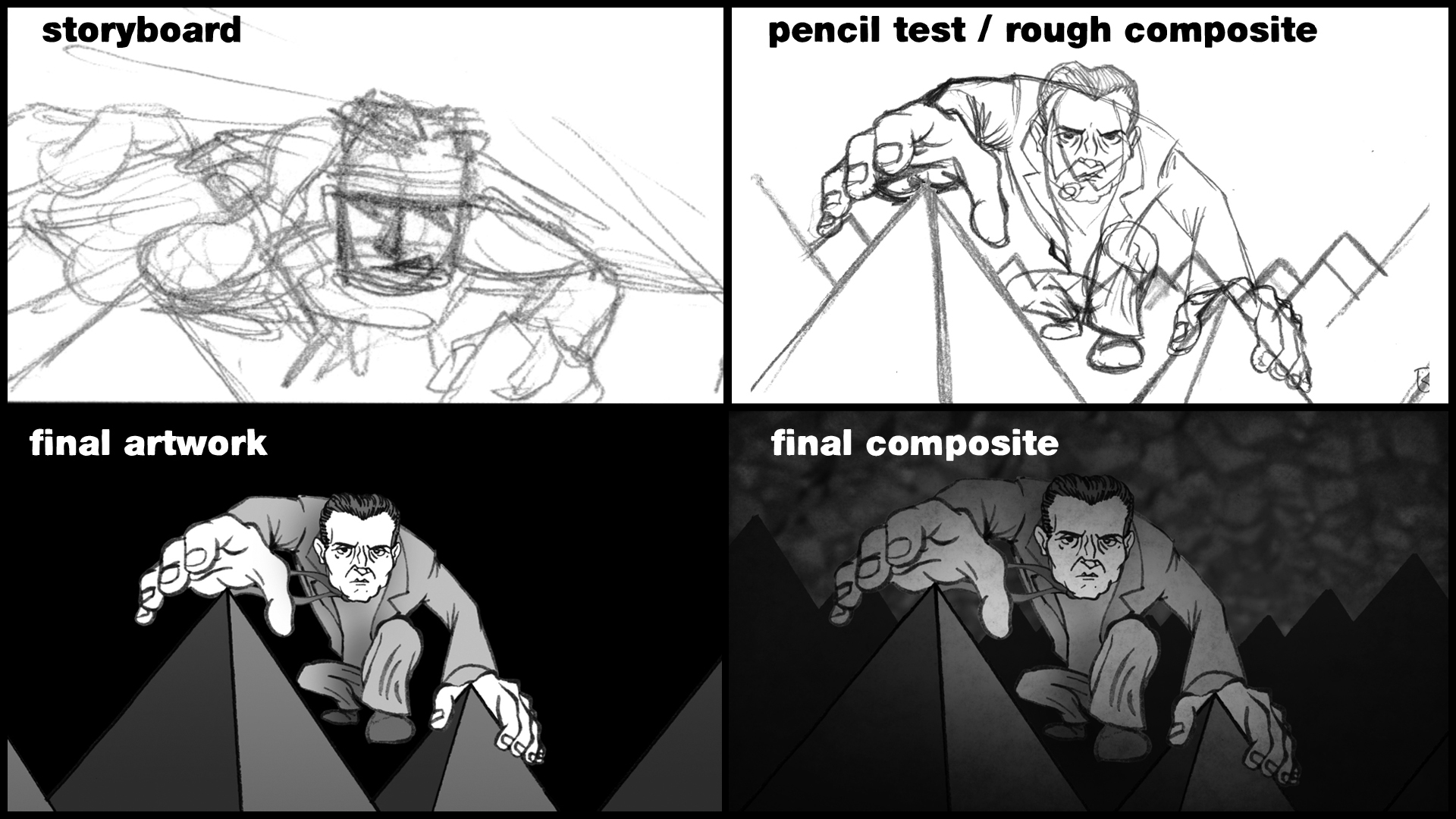
A look at the animation in progress.
What was your favorite part of working on this lesson? What was the hardest part?
AR: For me, my favorite part was working with these guys. I think it was a “the whole is greater than the sum of its parts” sort of situation. Writing with George, we have a very complementary skill set, and I think in passing the scripts back and forth, we were able to create something a lot stronger than what I would have been created on my own. And seeing what Jeremiah did with it was really wonderful and satisfying. The hardest part, I think, was struggling with the question, “Is the narrative overwhelming the educational material?” We put a lot into the subtext in the hope that people would get there watching it and thinking about it. I’m very curious to hear people’s impression of how it turned out.
GZ: My favorite thing is basically the same as Alex’s. I know for sure that working together we created a script that was far better than anything that I could’ve done myself. So that was a big part. The hardest part for me was the voiceover. I’m not a trained actor, by any stretch. My degree’s in chemistry. So going from the regular style of just, “I’m teaching something to you” to, “I’m totally hard-boiled and doing my best to solve the case of the missing triangle” was really difficult.
JD: Usually the funnest part of the job for me is figuring out how to visualize the lesson, but in this case George and Alex really had spelled a lot of it out already on the page. So, I really enjoyed making the most of the time I could spend working on the look and feel of it, and figuring out what constraints I would work within. I knew I wanted to do it in black and white, even the animation of the Mandlebrot set at the end, which you usually see rendered in all sorts of psychedelic colors. Of all the fractals, the Mandlebrot set was actually the easiest to animate, as the computer was able to do all the work—it just took several hours to render. The Sierpinski Triangle and the Koch Snowflake were the biggest challenges. I had to basically figure out and create one iteration of each transformation using 2D computer graphics and then composite or assemble a never-ending loop of the transformations happening for the whole of each fractal. It got more complicated when we had to zoom in to illustrate exactly what was happening. Still, just like doing the math involved in describing a piece of infinite geometry, all you had to do was get the one iteration right, and the whole motion graphic would seamlessly repeat itself when assembled properly. The hard part was just getting to that point.

A stack of Jeremiah Dickey’s drawings from the lesson.
This piece originally ran on the TED-Ed Blog. Check out lots more from our education-focused initiative:
Comments (7)